Musical Intervals: The Building Blocks of Music

b4n1
May 17, 2025, 4:26 p.m.
Musical Intervals: The Building Blocks of Music
Summary:
Musical intervals are the distances between two notes, constituting the fundamental element upon which melodies, harmonies, and scales are built. Understanding intervals is essential for any music student, as they represent the basic vocabulary with which all musical structures are articulated. This article explores their nature, classification, and application in music theory and practice.
Keywords:
Musical intervals, tones, semitones, consonance, dissonance, melody, harmony, ear training, music theory.
Introduction:
Imagine music as a language, where notes are letters and intervals are the syllables that give meaning and expression to that language. Just as we cannot form words without knowing how to combine letters, we cannot truly understand music without understanding intervals. Musical intervals are the distances between notes, measured in terms of tones and semitones.
The ability to recognize intervals aurally and understand their theoretical properties transforms our musical perception. A musician who masters intervals can transcribe melodies by ear, improvise fluently, and analyze compositions in depth. Moreover, this knowledge facilitates communication between musicians from different traditions and backgrounds, establishing common ground for creation and analysis.
Definition and Classification:
A musical interval is the distance in pitch between two notes. This distance is measured in degrees (according to position in the scale) and in tones and semitones (according to acoustic distance).
Intervals are classified in several ways:
According to their number of degrees:
- Unison: Same degree (Ex. C-C)
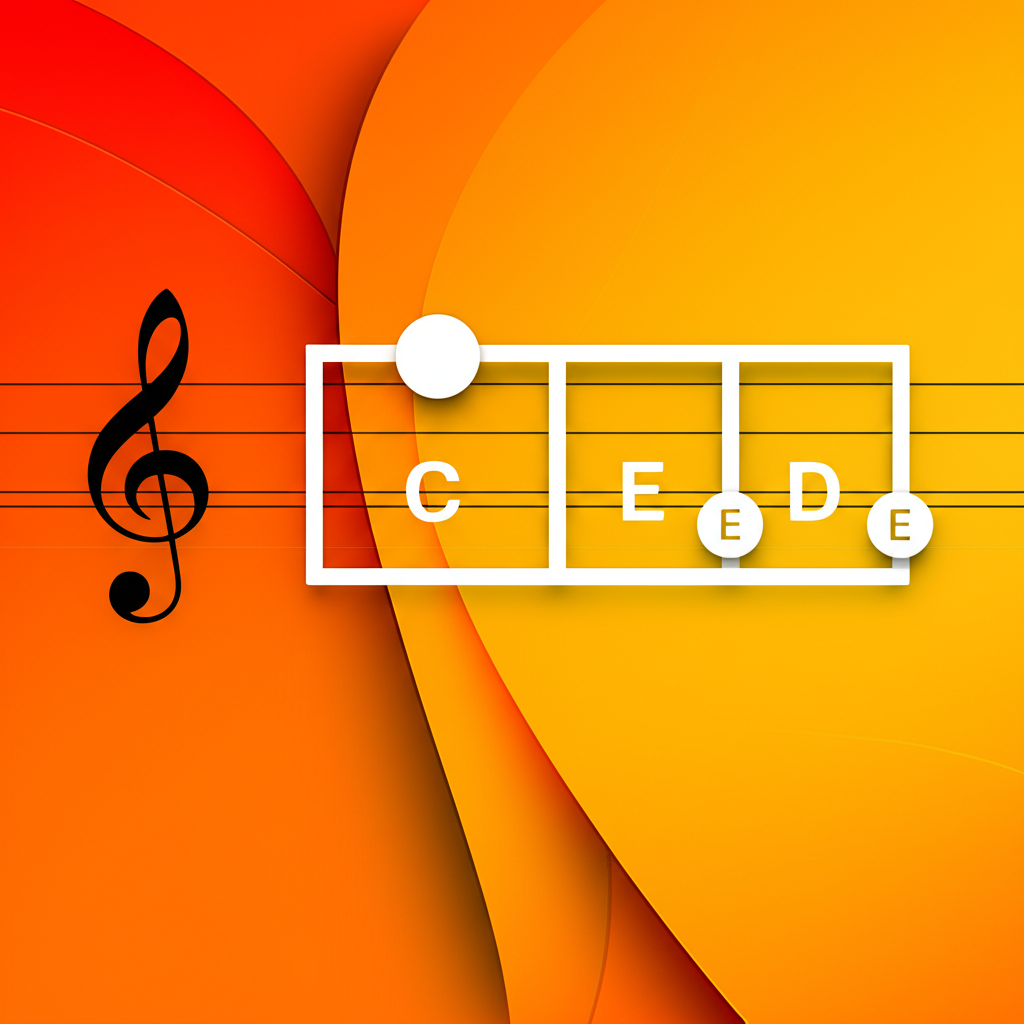
- Second: Two consecutive degrees (Ex. C-D)
- Third: Three degrees (Ex. C-E)
- Fourth: Four degrees (Ex. C-F)
- Fifth: Five degrees (Ex. C-G)
- Sixth: Six degrees (Ex. C-A)
- Seventh: Seven degrees (Ex. C-B)
- Octave: Eight degrees (Ex. C-C')
According to their quality:
- Major and minor: Applicable to seconds, thirds, sixths, and sevenths
- Perfect: Applicable to unisons, fourths, fifths, and octaves
- Augmented: Half step larger than major or perfect
- Diminished: Half step smaller than minor or perfect
According to their acoustic effect:
- Consonant: Produce a sense of stability (octaves, perfect fifths, major and minor thirds and sixths)
- Dissonant: Create tension (seconds, sevenths, augmented fourths/diminished fifths)
The 12 Intervals of the First Octave:
In the Western tempered system, we find 12 chromatic intervals in the first octave. Here we detail them with their main characteristics:
- Unison (0 semitones): Two identical notes played simultaneously. Example: C-C.
- Minor second (1 semitone): Dissonant interval that creates tension. Example: C-Db.
- Major second (2 semitones): Dissonant interval but more stable than the minor second. Example: C-D.
- Minor third (3 semitones): Consonant interval with a melancholic character. Example: C-Eb.
- Major third (4 semitones): Consonant interval with a bright or cheerful character. Example: C-E.
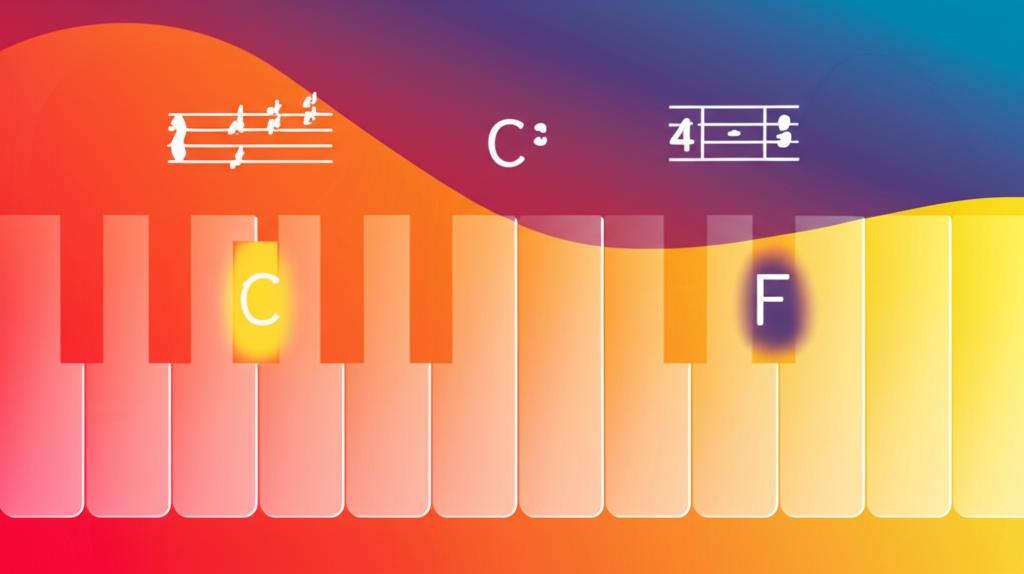
- Perfect fourth (5 semitones): Widely used consonant interval. Example: C-F.
- Tritone (6 semitones): Highly dissonant interval also known as augmented fourth (C-F#) or diminished fifth (C-Gb).
- Perfect fifth (7 semitones): Very consonant and stable interval. Example: C-G.
- Minor sixth (8 semitones): Consonant interval with a certain melancholic character. Example: C-Ab.
- Major sixth (9 semitones): Consonant interval with a luminous character. Example: C-A.
- Minor seventh (10 semitones): Dissonant interval with a strong tendency to resolve. Example: C-Bb.
- Major seventh (11 semitones): Very dissonant interval with an intense tendency to resolve. Example: C-B.
- Octave (12 semitones): Perfectly consonant interval, perceived as the same note in a different register. Example: C-C'.
Compound Intervals and Tensions:
Compound intervals are those that exceed the octave. These intervals are particularly important in modern harmony, especially in jazz and contemporary music, where they function as tensions that add color and expressiveness to chords.
Main Compound Intervals:
- Minor ninth (13 semitones): Minor second plus an octave. Creates dark tension in dominant chords. Example: C-Db'.
- Major ninth (14 semitones): Major second plus an octave. Adds brightness to major and dominant chords. Example: C-D'.
- Perfect eleventh (17 semitones): Perfect fourth plus an octave. Creates modal and suspended textures. Example: C-F'.
- Augmented eleventh (18 semitones): Tritone plus an octave. Essential in altered chords. Example: C-F#'.
- Minor thirteenth (20 semitones): Minor sixth plus an octave. Adds melancholic color. Example: C-Ab'.
- Major thirteenth (21 semitones): Major sixth plus an octave. Provides luminosity. Example: C-A'.
Applications of Compound Intervals as Tensions:
In modern harmony, these tensions enrich basic chords:
- Major chords: Major 9th, augmented 11th, and major 13th are available tensions.
- Minor chords: Major 9th, perfect 11th, and major 13th are common tensions.
- Dominant chords: Can incorporate all tensions (minor/major 9th, perfect/augmented 11th, minor/major 13th) depending on the harmonic context.
- Half-diminished chords: Major 9th and perfect 11th are characteristic tensions.
Examples:
Examples in ABC Notation: Each Interval in Treble Clef
Below is an example of each interval starting from F (in treble clef):
Compound Intervals in Treble Clef
Examples of compound intervals used as tensions in chords:
Harmonic Progressions:
Harmonic progressions are built by combining chords, which are themselves formed using intervals. Intervals not only determine how each chord sounds individually but also how music flows when chords succeed one another. In tonal music, movements of descending perfect fifth (or ascending perfect fourth) between chord roots are especially important for creating a sense of resolution.
From Intervals to Scales and Chords:
Musical intervals are the fundamental building blocks from which both scales and chords are constructed. Understanding how these elements relate is essential for mastering music theory.
Creating Scales through Intervals:
Scales are specific patterns of intervals. For example:
- Major Scale: Follows the interval pattern: M2-M2-m2-M2-M2-M2-m2
- Natural Minor Scale: Follows the pattern: M2-m2-M2-M2-m2-M2-M2
- Major Pentatonic Scale: Uses the pattern: M2-M2-m3-M2-m3
- Blues Scale: Combines: m3-M2-m2-m2-m3-M2
By applying these interval formulas to any starting note, we can construct the corresponding scale. For example, if we start with F and apply the major scale pattern (M2-M2-m2-M2-M2-M2-m2), we get: F-G-A-Bb-C-D-E-F.
Creating Chords through Intervals:
Chords are formed by stacking specific intervals:
- Major Chord: Root note + major third + perfect fifth (example: F-A-C)
- Minor Chord: Root note + minor third + perfect fifth (example: F-Ab-C)
- Augmented Chord: Root note + major third + augmented fifth (example: F-A-C#)
- Diminished Chord: Root note + minor third + diminished fifth (example: F-Ab-Cb)
- Dominant Seventh Chord: Major chord + minor seventh (example: F-A-C-Eb)
- Major Seventh Chord: Major chord + major seventh (example: F-A-C-E)
Harmonic Progressions:
Harmonic progressions are built by combining chords, which are themselves formed using intervals. Intervals not only determine how each chord sounds individually but also how music flows when chords succeed one another. In tonal music, movements of descending perfect fifth (or ascending perfect fourth) between chord roots are especially important for creating a sense of resolution.
Historical Figures:
Pythagoras (570-495 BC): Considered the father of musical interval theory, Pythagoras discovered the mathematical relationship between musical notes. Through experiments with a monochord, he determined that the most consonant intervals (octave, fifth, fourth) corresponded to simple mathematical ratios. His Pythagorean scale, based on perfect fifths, was fundamental to the development of Western music.
Jean-Philippe Rameau (1683-1764): This French composer and theorist revolutionized the understanding of intervals through his "Treatise on Harmony" (1722). Rameau proposed that consonances and dissonances were not arbitrary but based on natural acoustic phenomena such as the overtone series. His work established the principles of the modern tonal system, explaining how different intervals create tension and resolution in music.
Paul Hindemith (1895-1963): German composer who developed a comprehensive theory of intervals in his work "The Craft of Musical Composition" (1937). Hindemith proposed a hierarchy of intervals based on their acoustic stability, classifying them from most consonant to most dissonant. His approach influenced both 20th-century composition and contemporary music teaching methodologies.
Interesting Facts:
The interval called "tritone" (three whole tones, or augmented fourth/diminished fifth) was known in the Middle Ages as "diabolus in musica" (the devil in music) due to its dissonant sound and difficult intonation. Ironically, this interval, so avoided in medieval music, became an essential element of modern jazz and rock.
The human ear has a remarkable ability to recognize octaves. When we hear two notes separated by an octave, we tend to perceive them as "the same note" but at a different height. This phenomenon, known as "octave equivalence," is present in virtually all musical cultures around the world.
Intervals directly affect our emotions. Major thirds tend to be associated with joy, while minor thirds are often perceived as melancholic. This emotional response to intervals is surprisingly consistent among different listeners, even among those without musical training.
Microtones are intervals smaller than a semitone and are used in many non-Western musical traditions. Arabic music, for example, employs quarter-tone intervals, creating scales with more notes than the 12 in the Western system, allowing musical expressions impossible in our tempered system.
Conclusions:
Musical intervals are much more than simple distances between notes; they are the fundamental building blocks with which all musical experience is constructed. Their deep understanding opens doors to a richer appreciation of music, both as a listener and as a creator. From Pythagorean mathematical relationships to the complex harmonies of contemporary jazz, intervals remain the immutable alphabet of musical language.
As music students, training in interval recognition should be a constant practice, as it simultaneously develops ear training, theoretical understanding, and interpretive ability. Have you noticed how certain intervals evoke specific emotions? Or how certain musical genres favor particular intervals over others? These personal observations can be the starting point for a deeper exploration of this fascinating aspect of music.
References:
-
Kostka, S., & Payne, D. (2013). Tonal Harmony: With an Introduction to Twentieth-Century Music. McGraw-Hill Education.
-
Hindemith, P. (1942). The Craft of Musical Composition, Book I: Theory. Schott.
-
Levine, M. (1995). The Jazz Theory Book. Sher Music Co.
-
Burns, E. M., & Ward, W. D. (1978). Categorical perception of musical intervals. The Journal of the Acoustical Society of America, 63(2), 456-461.
-
Tenney, J. (1988). A History of 'Consonance' and 'Dissonance'. Excelsior Music Publishing Company.